This week’s simulation shows that the most likely range for the 3-month U.S. Treasury bill yield in ten years is from 0% to 1%. There is a 30.82% probability that the 3-month yield falls in this range, a change from 30.64% last week. For the 10-year Treasury yield, the most likely range is from 2% to 3%. The probability of being in this range is 27.48%, compared to 27.66% last week. How were these numbers derived? We explain that in this post.
In a recent post on SeekingAlpha, we pointed out that a forecast of “heads” or “tails” in a coin flip leaves out critical information.
https://seekingalpha.com/article/4437074-us-treasury-yields-the-10-year-probabilities
What a sophisticated bettor needs to know is that, on average for a fair coin, the probability of heads is 50%. A forecast that the next coin flip will be “heads” is literally worth nothing to investors because the outcome is purely random.
The same is true for interest rates.
In this post we present the probability distribution for both the 3-month Treasury bill rate and the 10-year U.S. Treasury yield 10 years forward using semi-annual time steps. We present the probability of where rates will be at each time step in 1 percent “rate buckets.” The forecast is shown in this graph:
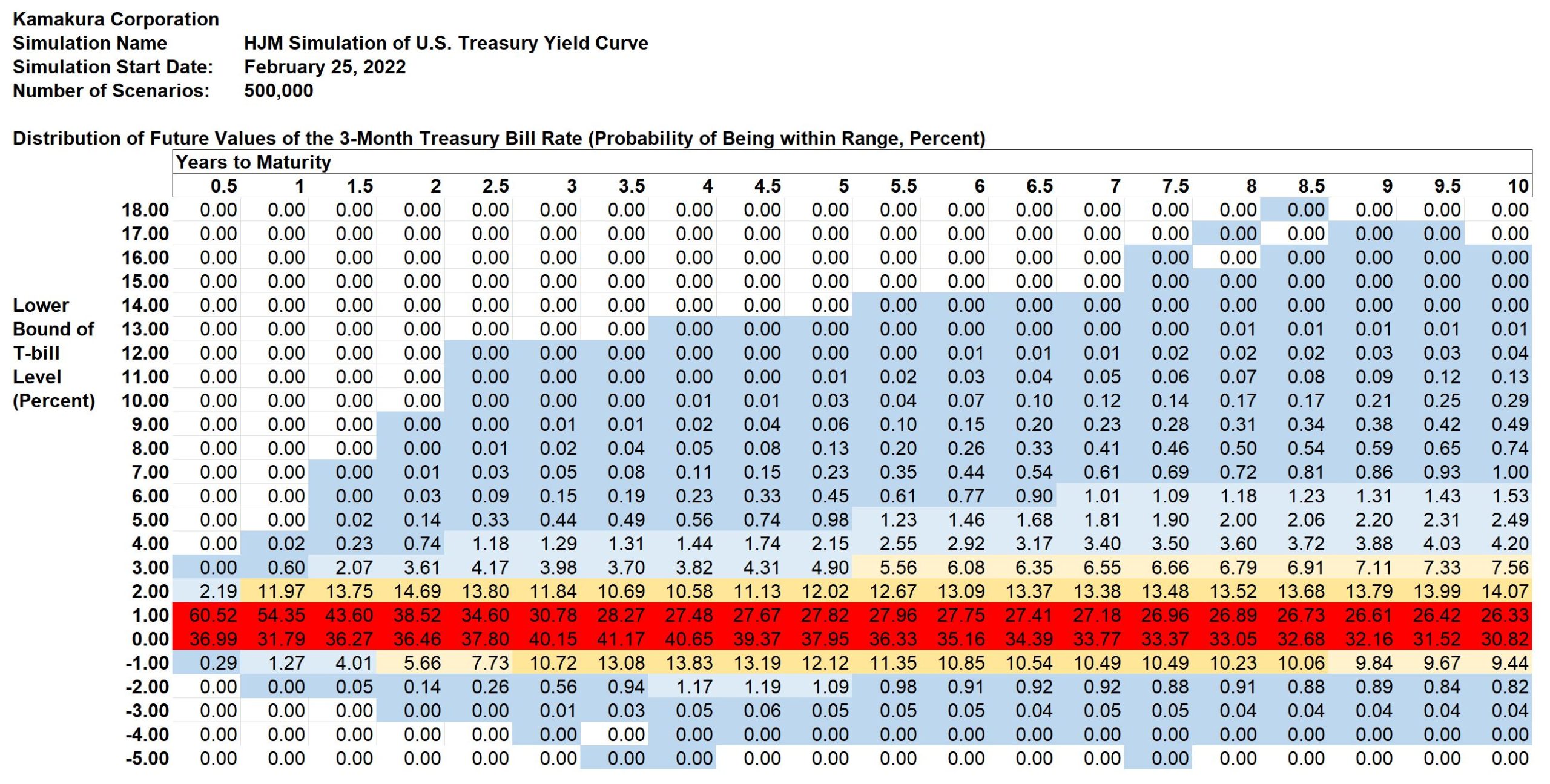
3-Month U.S. Treasury Yield Data:
The probability that the 3-month Treasury bill yield will be between 1% and 2% in 2 years is shown in column 4: 38.52%. The probability that the 3-month Treasury bill yield will be negative (as it has been often in Europe and Japan) in 2 years is 5.66% plus 0.14% plus 0.00% plus 0.00% = 5.80%, down from 6.66% last week. Cells shaded in blue represent positive probabilities of occurring, but the probability has been rounded to the nearest 0.01%. The shading scheme works like this:
Dark blue: the probability is greater than 0% but less than 1%
Light blue: the probability is greater than or equal to 1% and less than 5%
Light yellow: the probability is greater than or equal to 5% and 10%
Medium yellow: the probability is greater than or equal to 10% and less than 20%
Orange: the probability is greater than or equal to 20% and less than 25%
Red: the probability is greater than 25%
The chart below shows the same probabilities for the 10-year U.S. Treasury yield derived as part of the same simulation.

10-Year US Treasury Yield Data:
How are the Probabilities Derived?
The probabilities are derived using the same methodology that Kamakura recommends to its risk management clients, who currently have more than $38 trillion in assets or assets under management. A moderately technical explanation is given in the appendix, but we summarize it in plain English here:
Step 1: We take the closing U.S. Treasury yield curve as our starting point. For today’s forecast that’s February 25, 2022.
Step 2: We use the number of points on the yield curve that best explain historical yield curve shifts. Using daily data from 1962 through December 31, 2021, that’s 10 “factors.”
Step 3: We measure the volatility of changes in those factors and how it has changed over the same period.
Step 4: Using those measured volatilities, we generate 500,000 random shocks at each time step and derive the resulting yield curve.
Step 5: We “validate” the model to make sure that the simulation EXACTLY prices the starting Treasury curve and that it fits history as well as possible. The methodology for doing this is described in the appendix.
Step 6: We take all 500,000 simulated yield curves and calculate the probabilities that yields fall in each of the 1% “buckets” displayed in the graph.
Should I buy a long term bond or a short term bond?
We showed in a recent post on SeekingAlpha that, on average, investors have almost always done better by buying long term bonds than by rolling over short term Treasury bills.
https://seekingalpha.com/article/4480279-how-well-do-us-treasury-yields-forecast-inflation
That means that market participants have generally (but not always) been accurate in forecasting future inflation and adding a risk premium to that forecast.
The distribution above helps investors estimate the probability of success from going long.
Finally, as we remind readers weekly in The Corporate Bond Investor Friday overview, the future expenses (both the amount and the timing) that all investors are trying to cover with their investments are an important part of investment strategy. The author follows his own advice: cover the short-term cash needs first and then step out to cover more distant cash needs as savings and investment returns accumulate.
Please let us know if you have any comments, questions or suggestions.
Technical Appendix
Daily Treasury yields form the base historical data for fitting the number of yield curve factors and their volatility. We used daily data from January 2, 1962 through December 31, 2021 for today’s forecast. The historical data is provided by the U.S. Department of the Treasury.
An example of the modeling process using data through December 31, 2021 is available at this link:
The modeling process was published in a very important paper by David Heath, Robert Jarrow and Andrew Morton in 1992:

Link for the paper:
https://ideas.repec.org/a/ecm/emetrp/v60y1992i1p77-105.html
Professor Jarrow’s biography:
https://www.kamakuraco.com/company/executive-profiles/robert-a-jarrow-ph-d/
For technically inclined readers, we recommend this book by Prof. Jarrow for those who want to know exactly how the “HJM” model construction works:
The number of factors (10 for the United States) has been stable for some time, but the volatilities for each factor are updated monthly and available to subscribers to the KRIS interest rate and macro-factor data service:
The number of scenarios used for today’s simulation was 500,000.


